अर्धसंक्रमणीय संबंध: Difference between revisions
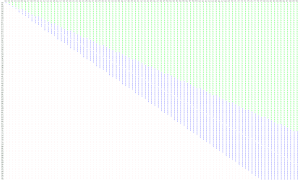
(Created page with "File:Quasitransitive 25 percent margin.gif|thumb|अर्धसंक्रमणीय संबंध x≤{{sfrac|5|4}}य. इसका सममित और सकर...") |
No edit summary |
||
| (3 intermediate revisions by 3 users not shown) | |||
| Line 1: | Line 1: | ||
[[File:Quasitransitive 25 percent margin.gif|thumb|अर्धसंक्रमणीय संबंध x≤{{sfrac|5|4}} | [[File:Quasitransitive 25 percent margin.gif|thumb|अर्धसंक्रमणीय संबंध x≤{{sfrac|5|4}}y इसका सममित और सकर्मक भाग क्रमशः नीले और हरे रंग में दिखाया गया है।]]'''अर्धसंक्रमणीय''' की गणितीय धारणा संक्रमणीय संबंध का शक्तिहीन संस्करण है जिसका उपयोग सामाजिक विकल्प सिद्धांत और सूक्ष्मअर्थशास्त्र में किया जाता है। अनौपचारिक रूप से, संबंध अर्धसंक्रमणीय होता है यदि यह कुछ मानों के लिए [[सममित संबंध]] हो और अन्यत्र सकर्मक हो। एरो प्रमेय के परिणामों का अध्ययन करने के लिए इस अवधारणा को {{harvtxt|सेन|1969}} (1969) द्वारा प्रस्तुत किया गया था। | ||
==औपचारिक परिभाषा== | ==औपचारिक परिभाषा== | ||
समुच्चय (गणित) | |||
: <math>(a\operatorname{T}b) \wedge \neg(b\operatorname{T}a) \wedge (b\operatorname{T}c) \wedge \neg(c\operatorname{T}b) \Rightarrow (a\operatorname{T}c) \wedge \neg(c\operatorname{T}a).</math> | : <math>(a\operatorname{T}b) \wedge \neg(b\operatorname{T}a) \wedge (b\operatorname{T}c) \wedge \neg(c\operatorname{T}b) \Rightarrow (a\operatorname{T}c) \wedge \neg(c\operatorname{T}a).</math> | ||
यदि संबंध भी [[एंटीसिमेट्रिक संबंध]] है, तो T सकर्मक है। | यदि संबंध भी [[एंटीसिमेट्रिक संबंध|प्रतिसममितीय संबंध]] है, तो T सकर्मक है। | ||
वैकल्पिक रूप से, किसी संबंध | वैकल्पिक रूप से, किसी संबंध T के लिए, [[असममित संबंध]] या जटिल भाग P को परिभाषित करें: | ||
:<math>(a\operatorname{P}b) \Leftrightarrow (a\operatorname{T}b) \wedge \neg(b\operatorname{T}a).</math> | :<math>(a\operatorname{P}b) \Leftrightarrow (a\operatorname{T}b) \wedge \neg(b\operatorname{T}a).</math> | ||
तब T अर्धसंक्रमणीय है यदि | तब T अर्धसंक्रमणीय है यदि केवल P सकर्मक है। | ||
==उदाहरण== | ==उदाहरण== | ||
कुछ आर्थिक संदर्भों में प्राथमिकताओं को अर्धसंक्रमणीय (संक्रमणीय के | कुछ आर्थिक संदर्भों में प्राथमिकताओं को अर्धसंक्रमणीय (संक्रमणीय के अतिरिक्त) माना जाता है। क्लासिक उदाहरण एक व्यक्ति है जो 7 और 8 ग्राम चीनी के मध्य उदासीन है 8 और 9 ग्राम चीनी के मध्य उदासीन है, किंतु जो 7 की तुलना में 9 ग्राम चीनी में [[पसंद|रूचि]] रखता है।<ref>{{cite journal | url=https://www.imbs.uci.edu/files/personnel/luce/pre1990/1956/Luce_Econometrica_1956.pdf | author=Robert Duncan Luce | author-link=Robert Duncan Luce | title=अर्धआदेश और उपयोगिता भेदभाव का एक सिद्धांत| journal=Econometrica | volume=24 | number=2 | pages=178–191 | date=Apr 1956 | doi=10.2307/1905751| jstor=1905751 }} Here: p.179; Luce's original example consists in 400 comparisons (of coffee cups with different amounts of sugar) rather than just 2.</ref> इसी प्रकार, सोराइट्स विरोधाभास को अर्धसंक्रमणीय के कुछ संबंधों की अनुमानित परिवर्तनशीलता को शक्तिहीन करके समाधान किया जा सकता है। | ||
==गुण== | ==गुण== | ||
* | * संबंध R अर्धसंक्रमणीय है यदि, केवल यह सममित संबंध J और संक्रमणीय संबंध P का [[असंयुक्त संघ]] है।<ref>The naminig follows {{harvtxt|Bossert|Suzumura|2009}}, p.2-3.<!---'J' is used instead of 'I' to increase readability with sans-serif fonts---> — For the ''only-if'' part, define ''xJy'' as ''xRy'' ∧ ''yRx'', and define ''xPy'' as ''xRy'' ∧ ¬''yRx''. — For the ''if'' part, assume ''xRy'' ∧ ¬''yRx'' ∧ ''yRz'' ∧ ¬''zRy'' holds. Then ''xPy'' and ''yPz'', since ''xJy'' or ''yJz'' would contradict ¬''yRx'' or ¬''zRy''. Hence ''xPz'' by transitivity, ¬''xJz'' by disjointness, ¬''zJx'' by symmetry. Therefore, ''zRx'' would imply ''zPx'', and, by transitivity, ''zPy'', which contradicts ¬''zRy''. Altogether, this proves ''xRz'' ∧ ¬''zRx''.</ref>J और P किसी दिए गए R द्वारा विशिष्ट रूप से निर्धारित नहीं होते हैं;<ref>For example, if ''R'' is an [[equivalence relation]], ''J'' may be chosen as the [[empty relation]], or as ''R'' itself, and ''P'' as its complement.</ref> चूँकि, केवल-यदि भाग से P न्यूनतम है।<ref>Given ''R'', whenever ''xRy'' ∧ ¬''yRx'' holds, the pair (''x'',''y'') can't belong to the symmetric part, but must belong to the transitive part.</ref> | ||
* परिणामस्वरूप, प्रत्येक सममित संबंध अर्धसंक्रमणीय है, और इसी प्रकार प्रत्येक सकर्मक संबंध भी है।<ref>Since the empty relation is trivially both transitive and symmetric.</ref> इसके | * परिणामस्वरूप, प्रत्येक सममित संबंध अर्धसंक्रमणीय है, और इसी प्रकार प्रत्येक सकर्मक संबंध भी है।<ref>Since the empty relation is trivially both transitive and symmetric.</ref> इसके अतिरिक्त, एंटीसिमेट्रिक और अर्धसंक्रमणीय संबंध सदैव सकर्मक होता है।<ref>The antisymmetry of ''R'' forces ''J'' to be [[coreflexive relation|coreflexive]]; hence the union of ''J'' and the transitive ''P'' is again transitive.</ref> | ||
* उपरोक्त चीनी उदाहरण से संबंध, {(7,7), (7,8), (7,9), (8,7), (8,8), (8,9), (9,8 ), (9,9)}, अर्धसंक्रमणीय है, | * उपरोक्त चीनी उदाहरण से संबंध, {(7,7), (7,8), (7,9), (8,7), (8,8), (8,9), (9,8 ), (9,9)}, अर्धसंक्रमणीय है, किंतु सकर्मक नहीं है। | ||
* | * अर्धसंक्रमणीय संबंध को [[चक्रीय संबंध]] होने की आवश्यकता नहीं है: प्रत्येक अरिक्त सेट A के लिए, [[सार्वभौमिक संबंध]] A×A चक्रीय और अर्धसंक्रमणीय दोनों है। | ||
* कोई संबंध अर्धसंक्रमणीय है यदि | * कोई संबंध अर्धसंक्रमणीय है यदि केवल जब [[पूरक संबंध]] है। | ||
* इसी प्रकार, कोई संबंध अर्धसंक्रमणीय होता है यदि | * इसी प्रकार, कोई संबंध अर्धसंक्रमणीय होता है यदि इसका [[विपरीत संबंध]] हो। | ||
==यह भी देखें== | ==यह भी देखें== | ||
| Line 39: | Line 39: | ||
* {{cite book | title=Consistency, choice and rationality | first1=Walter | last1=Bossert | first2=Kōtarō | last2=Suzumura | publisher=Harvard University Press | year=2010 | isbn=978-0674052994 }} | * {{cite book | title=Consistency, choice and rationality | first1=Walter | last1=Bossert | first2=Kōtarō | last2=Suzumura | publisher=Harvard University Press | year=2010 | isbn=978-0674052994 }} | ||
* {{cite report | url=http://econ.haifa.ac.il/~admiller/ArrowWithoutTransitivity.pdf | author=Alan D. Miller and Shiran Rachmilevitch | title=Arrow's Theorem Without Transitivity | institution=University of Haifa | type=Working paper | date=Feb 2014 }} | * {{cite report | url=http://econ.haifa.ac.il/~admiller/ArrowWithoutTransitivity.pdf | author=Alan D. Miller and Shiran Rachmilevitch | title=Arrow's Theorem Without Transitivity | institution=University of Haifa | type=Working paper | date=Feb 2014 }} | ||
[[Category:Created On 30/06/2023]] | [[Category:Created On 30/06/2023]] | ||
[[Category:Machine Translated Page]] | |||
[[Category:Pages with script errors]] | |||
[[Category:Templates Vigyan Ready]] | |||
[[Category:द्विआधारी संबंध]] | |||
[[Category:सामाजिक चयन सिद्धांत]] | |||
Latest revision as of 09:30, 12 July 2023
अर्धसंक्रमणीय की गणितीय धारणा संक्रमणीय संबंध का शक्तिहीन संस्करण है जिसका उपयोग सामाजिक विकल्प सिद्धांत और सूक्ष्मअर्थशास्त्र में किया जाता है। अनौपचारिक रूप से, संबंध अर्धसंक्रमणीय होता है यदि यह कुछ मानों के लिए सममित संबंध हो और अन्यत्र सकर्मक हो। एरो प्रमेय के परिणामों का अध्ययन करने के लिए इस अवधारणा को सेन (1969) (1969) द्वारा प्रस्तुत किया गया था।
औपचारिक परिभाषा
समुच्चय (गणित)
यदि संबंध भी प्रतिसममितीय संबंध है, तो T सकर्मक है।
वैकल्पिक रूप से, किसी संबंध T के लिए, असममित संबंध या जटिल भाग P को परिभाषित करें:
तब T अर्धसंक्रमणीय है यदि केवल P सकर्मक है।
उदाहरण
कुछ आर्थिक संदर्भों में प्राथमिकताओं को अर्धसंक्रमणीय (संक्रमणीय के अतिरिक्त) माना जाता है। क्लासिक उदाहरण एक व्यक्ति है जो 7 और 8 ग्राम चीनी के मध्य उदासीन है 8 और 9 ग्राम चीनी के मध्य उदासीन है, किंतु जो 7 की तुलना में 9 ग्राम चीनी में रूचि रखता है।[1] इसी प्रकार, सोराइट्स विरोधाभास को अर्धसंक्रमणीय के कुछ संबंधों की अनुमानित परिवर्तनशीलता को शक्तिहीन करके समाधान किया जा सकता है।
गुण
- संबंध R अर्धसंक्रमणीय है यदि, केवल यह सममित संबंध J और संक्रमणीय संबंध P का असंयुक्त संघ है।[2]J और P किसी दिए गए R द्वारा विशिष्ट रूप से निर्धारित नहीं होते हैं;[3] चूँकि, केवल-यदि भाग से P न्यूनतम है।[4]
- परिणामस्वरूप, प्रत्येक सममित संबंध अर्धसंक्रमणीय है, और इसी प्रकार प्रत्येक सकर्मक संबंध भी है।[5] इसके अतिरिक्त, एंटीसिमेट्रिक और अर्धसंक्रमणीय संबंध सदैव सकर्मक होता है।[6]
- उपरोक्त चीनी उदाहरण से संबंध, {(7,7), (7,8), (7,9), (8,7), (8,8), (8,9), (9,8 ), (9,9)}, अर्धसंक्रमणीय है, किंतु सकर्मक नहीं है।
- अर्धसंक्रमणीय संबंध को चक्रीय संबंध होने की आवश्यकता नहीं है: प्रत्येक अरिक्त सेट A के लिए, सार्वभौमिक संबंध A×A चक्रीय और अर्धसंक्रमणीय दोनों है।
- कोई संबंध अर्धसंक्रमणीय है यदि केवल जब पूरक संबंध है।
- इसी प्रकार, कोई संबंध अर्धसंक्रमणीय होता है यदि इसका विपरीत संबंध हो।
यह भी देखें
संदर्भ
- ↑ Robert Duncan Luce (Apr 1956). "अर्धआदेश और उपयोगिता भेदभाव का एक सिद्धांत" (PDF). Econometrica. 24 (2): 178–191. doi:10.2307/1905751. JSTOR 1905751. Here: p.179; Luce's original example consists in 400 comparisons (of coffee cups with different amounts of sugar) rather than just 2.
- ↑ The naminig follows Bossert & Suzumura (2009), p.2-3. — For the only-if part, define xJy as xRy ∧ yRx, and define xPy as xRy ∧ ¬yRx. — For the if part, assume xRy ∧ ¬yRx ∧ yRz ∧ ¬zRy holds. Then xPy and yPz, since xJy or yJz would contradict ¬yRx or ¬zRy. Hence xPz by transitivity, ¬xJz by disjointness, ¬zJx by symmetry. Therefore, zRx would imply zPx, and, by transitivity, zPy, which contradicts ¬zRy. Altogether, this proves xRz ∧ ¬zRx.
- ↑ For example, if R is an equivalence relation, J may be chosen as the empty relation, or as R itself, and P as its complement.
- ↑ Given R, whenever xRy ∧ ¬yRx holds, the pair (x,y) can't belong to the symmetric part, but must belong to the transitive part.
- ↑ Since the empty relation is trivially both transitive and symmetric.
- ↑ The antisymmetry of R forces J to be coreflexive; hence the union of J and the transitive P is again transitive.
- Sen, A. (1969). "Quasi-transitivity, rational choice and collective decisions". Rev. Econ. Stud. 36 (3): 381–393. doi:10.2307/2296434. JSTOR 2296434. Zbl 0181.47302.
- Frederic Schick (Jun 1969). "Arrow's Proof and the Logic of Preference". Philosophy of Science. 36 (2): 127–144. doi:10.1086/288241. JSTOR 186166. S2CID 121427121.
- Amartya K. Sen (1970). Collective Choice and Social Welfare. Holden-Day, Inc.
- Amartya K. Sen (Jul 1971). "Choice Functions and Revealed Preference" (PDF). The Review of Economic Studies. 38 (3): 307–317. doi:10.2307/2296384. JSTOR 2296384.
- A. Mas-Colell and H. Sonnenschein (1972). "General Possibility Theorems for Group Decisions" (PDF). The Review of Economic Studies. 39 (2): 185–192. doi:10.2307/2296870. JSTOR 2296870. S2CID 7295776. Archived from the original (PDF) on 2018-04-12.
- D.H. Blair and R.A. Pollak (1982). "Acyclic Collective Choice Rules". Econometrica. 50 (4): 931–943. doi:10.2307/1912770. JSTOR 1912770.
- Bossert, Walter; Suzumura, Kotaro (Apr 2005). Rational Choice on Arbitrary Domains: A Comprehensive Treatment (PDF) (Technical Report). Université de Montréal, Hitotsubashi University Tokyo.
- Bossert, Walter; Suzumura, Kotaro (Mar 2009). Quasi-transitive and Suzumura consistent relations (PDF) (Technical Report). Université de Montréal, Waseda University Tokyo. doi:10.1007/s00355-011-0600-z. S2CID 38375142. Archived from the original (PDF) on 2018-04-12.
- Bossert, Walter; Suzumura, Kōtarō (2010). Consistency, choice and rationality. Harvard University Press. ISBN 978-0674052994.
- Alan D. Miller and Shiran Rachmilevitch (Feb 2014). Arrow's Theorem Without Transitivity (PDF) (Working paper). University of Haifa.