पॉलीट्रोपिक प्रक्रिया

"पैंट्रोपिक" redirects here. For बायोग्राफी में प्रयुक्त शब्द, see पैंट्रोपिकल.

"पॉलीट्रोपिक" redirects here. For वायरस में पॉलीट्रोपिज्म, see एम्फोट्रोपिज्म.

एक पॉलीट्रॉपिक प्रक्रिया एक थर्मोडायनामिक प्रक्रिया है जो संबंध का पालन करती है:

$$pV^{n}=C$$

जहाँ p दाब है, V आयतन है, n 'पॉलीट्रोपिक इंडेक्स' है, और C एक स्थिरांक है। पॉलीट्रोपिक प्रक्रिया समीकरण विस्तार और संपीड़न प्रक्रियाओं का वर्णन करता है जिसमें ऊष्मा स्थानांतरण शामिल है।

विशेष स्थितियां

n के कुछ विशिष्ट मूल्य विशेष स्थितियों के अनुरूप हैं:

• $n=0$ समदाब रेखीय प्रक्रिया के लिए,
• $n=+\infty$ एक आइसोकोरिक प्रक्रिया के लिए।

इसके अतिरिक्त, जब आदर्श गैस नियम लागू होता है:

• $n=1$ एक समतापी प्रक्रिया के लिए,
• $n=\gamma$ एक आइसेंट्रोपिक प्रक्रिया के लिए।

जहाँ $\gamma$ स्थिर दबाव पर ताप क्षमता का अनुपात है ($C_{P}$) स्थिर आयतन पर क्षमता को गर्म करने के लिए ($C_{V}$).

पॉलीट्रोपिक गुणांक और ऊर्जा स्थानांतरण के अनुपात के बीच समानता

पॉलीट्रॉपिक प्रक्रियाएं विभिन्न पॉलीट्रॉपिक इंडेक्स के साथ अलग-अलग व्यवहार करती हैं। एक पॉलीट्रॉपिक प्रक्रिया अन्य बुनियादी थर्मोडायनामिक प्रक्रियाओं को उत्पन्न कर सकती है।

गतिज ऊर्जा और स्थितिज ऊर्जा में नगण्य परिवर्तन के साथ एक धीमी प्रक्रिया से गुजरने वाली एक संवृत प्रणाली में एक आदर्श गैस के लिए प्रक्रिया पॉलीट्रोपिक है, जैसे कि

$$pv^{(1-\gamma )K+\gamma }=C$$

जहां C स्थिर है, $K={\frac {\delta q}{\delta w}}$, $\gamma ={\frac {c_{p}}{c_{v}}}$, और पॉलीट्रोपिक गुणांक के साथ $n={(1-\gamma )K+\gamma }$.

आदर्श प्रक्रियाओं से संबंध

पॉलीट्रोपिक इंडेक्स के कुछ मूल्यों के लिए, प्रक्रिया अन्य सामान्य प्रक्रियाओं का पर्याय बन जाएगी। विभिन्न सूचकांक मूल्यों के प्रभावों के कुछ उदाहरण निम्न तालिका में दिए गए हैं।

Variation of polytropic index n

Polytropic index	Relation	Effects
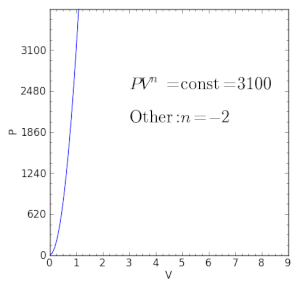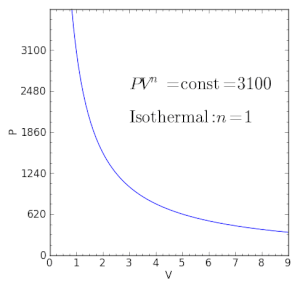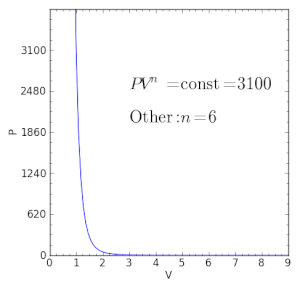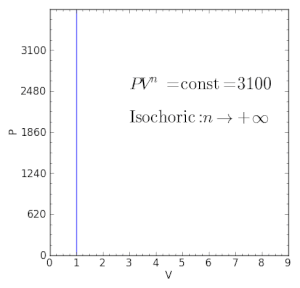
n < 0	—	Negative exponents reflect a process where work and heat flow simultaneously in or out of the system. In the absence of forces except pressure, such a spontaneous process is not allowed by the second law of thermodynamics; however, negative exponents can be meaningful in some special cases not dominated by thermal interactions, such as in the processes of certain plasmas in astrophysics, or if there are other forms of energy (e.g. chemical energy) involved during the process (e.g. explosion).
n = 0	$p=C$	Equivalent to an isobaric process (constant pressure)
n = 1	$pV=C$	Equivalent to an isothermal process (constant temperature), under the assumption of ideal gas law, since then $pV=nRT$.
1 < n < γ	—	Under the assumption of ideal gas law, heat and work flows go in opposite directions (K > 0), such as in vapor compression refrigeration during compression, where the elevated vapour temperature resulting from the work done by the compressor on the vapour leads to some heat loss from the vapour to the cooler surroundings.
n = γ	—	Equivalent to an isentropic process (adiabatic and reversible, no heat transfer), under the assumption of ideal gas law.
γ < n < ∞	—	Under the assumption of ideal gas law, heat and work flows go in the same direction (K < 0), such as in an internal combustion engine during the power stroke, where heat is lost from the hot combustion products, through the cylinder walls, to the cooler surroundings, at the same time as those hot combustion products push on the piston.
n = +∞	$V=C$	Equivalent to an isochoric process (constant volume)

जब इंडेक्स एन पूर्व मानों (0, 1, γ, या ∞) में से किसी दो के बीच होता है, तो इसका मतलब है कि पॉलीट्रॉपिक वक्र दो बाध्यकारी सूचकांकों के घटता (परिबद्ध समारोह) के माध्यम से कट जाएगा।

एक आदर्श गैस के लिए, 1 < γ < 5/3, चूंकि मेयर के संबंध से

$$\gamma ={\frac {c_{p}}{c_{v}}}={\frac {c_{v}+R}{c_{v}}}=1+{\frac {R}{c_{v}}}={\frac {c_{p}}{c_{p}-R}}.$$

अन्य

बहुरूपीिक तरल पदार्थ का उपयोग करके लेन-एम्डेन समीकरण का समाधान एक पॉलीट्रॉप के रूप में जाना जाता है।

यह भी देखें

• एडियाबेटिक प्रक्रिया
• कंप्रेसर
• आंतरिक दहन इंजन
• आइसेंट्रोपिक प्रक्रिया
• आइसोबैरिक प्रक्रिया
• आइसोकोरिक प्रक्रिया
• इज़ोटेर्मल प्रक्रिया
• पॉलीट्रोप
• क्वासिस्टैटिक संतुलन
• ऊष्मप्रवैगिकी
• वाष्प-संपीड़न प्रशीतन